New

HW Exam IV

Assigned Monday 04/27/20, due Monday 05/04/20

Introduction

In room temperature plasticity deformation was induced by the movement of dislocations. The dislocations were defined by a slip vector and a slip plane. The movement of dislocations in the slip plane caused the upper half of the crystal to slide over the lower half by a distance equal to the slip vector, which was a lattice translation vector. To repeat, plastcity involves collective movement of atoms on the scale of dislocation spacing, which can be several micrometers.

Deformation at high temperature is achieved by the movement of single atoms. The atoms move in discreet jumps to produce a change in the shape of a polycrystal. A polycrystal is an aggregate of small "grains" or crystallites that form grain boundaries with their neighbors. Grain boundaries play a critical role in this mechanism by serving as the source and the sink for atoms: for example atoms can be etched at a grain boundary in compression and transported by diffusion to the "orthogonal" grain boundary which is under tension. In this way the grain becomes longer in the diretion of the tensile stress, and shorter under compressive stress. Deformation occurs at constant volume since mass must be conserved.

We shall study such "diffusional defomation" in the following steps (i) the geometry of the physical strain that is obtained by the transport of atoms between grain boundaries in compressio and temsion, (ii) the elemental structure of grain boundaries that permits them to serve as both as a source and a sink of atoms, (iii) the fundamental descripion of diffusion of atoms - the diffusion coefficient, (iv) the translation of mechanical driving forces into a "chemical" driving force for diffusional transport in the direction from grain boundaries in compression to those in tension, and finally (v) derivation of a equation for the strain rate of a polycrystal under uniaxial load in terms of temperature, the applied stress and the grain size.

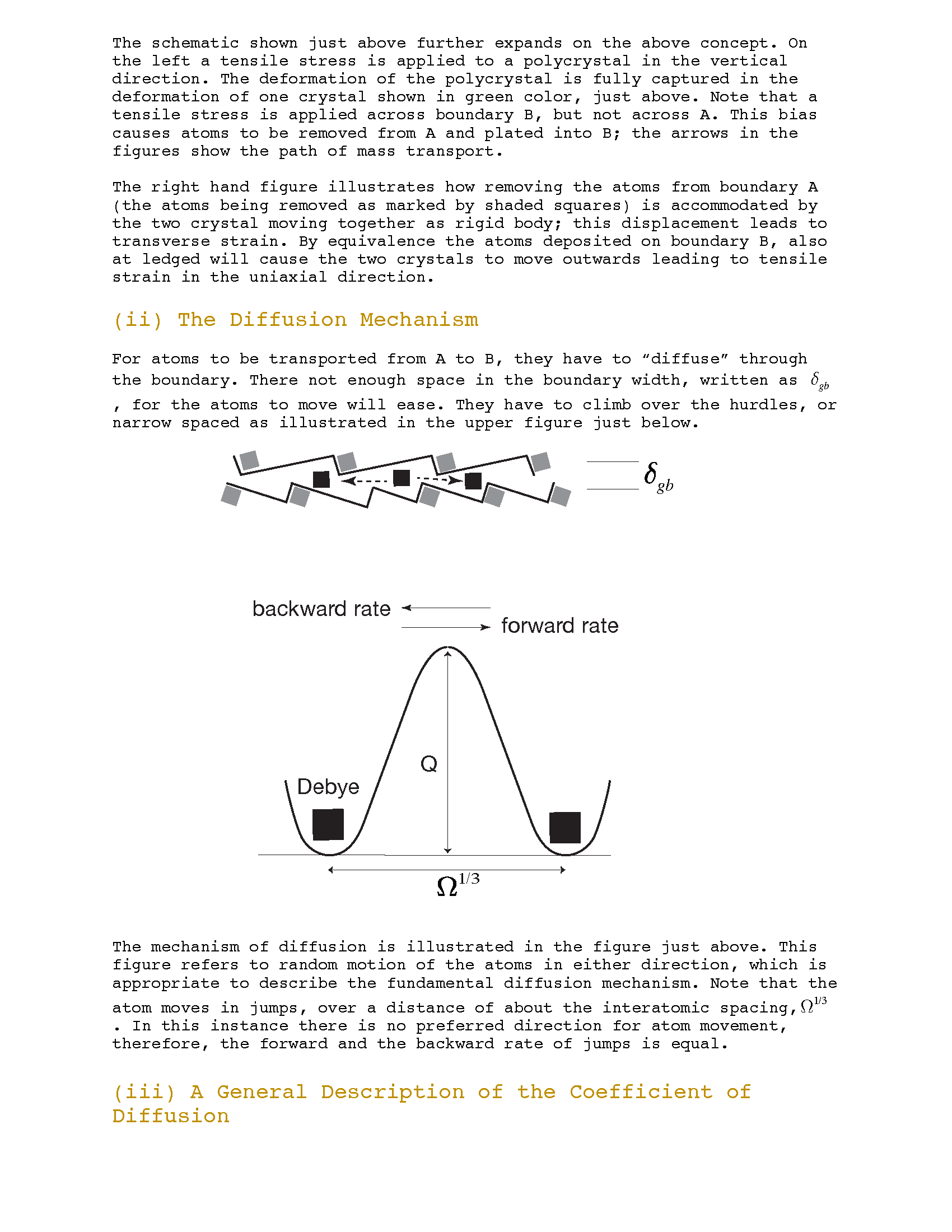
It is quite remarkable that very large deformations, so called superplasticity, is possible by such atom-wise transport of mass, as illustrated by the figure just below

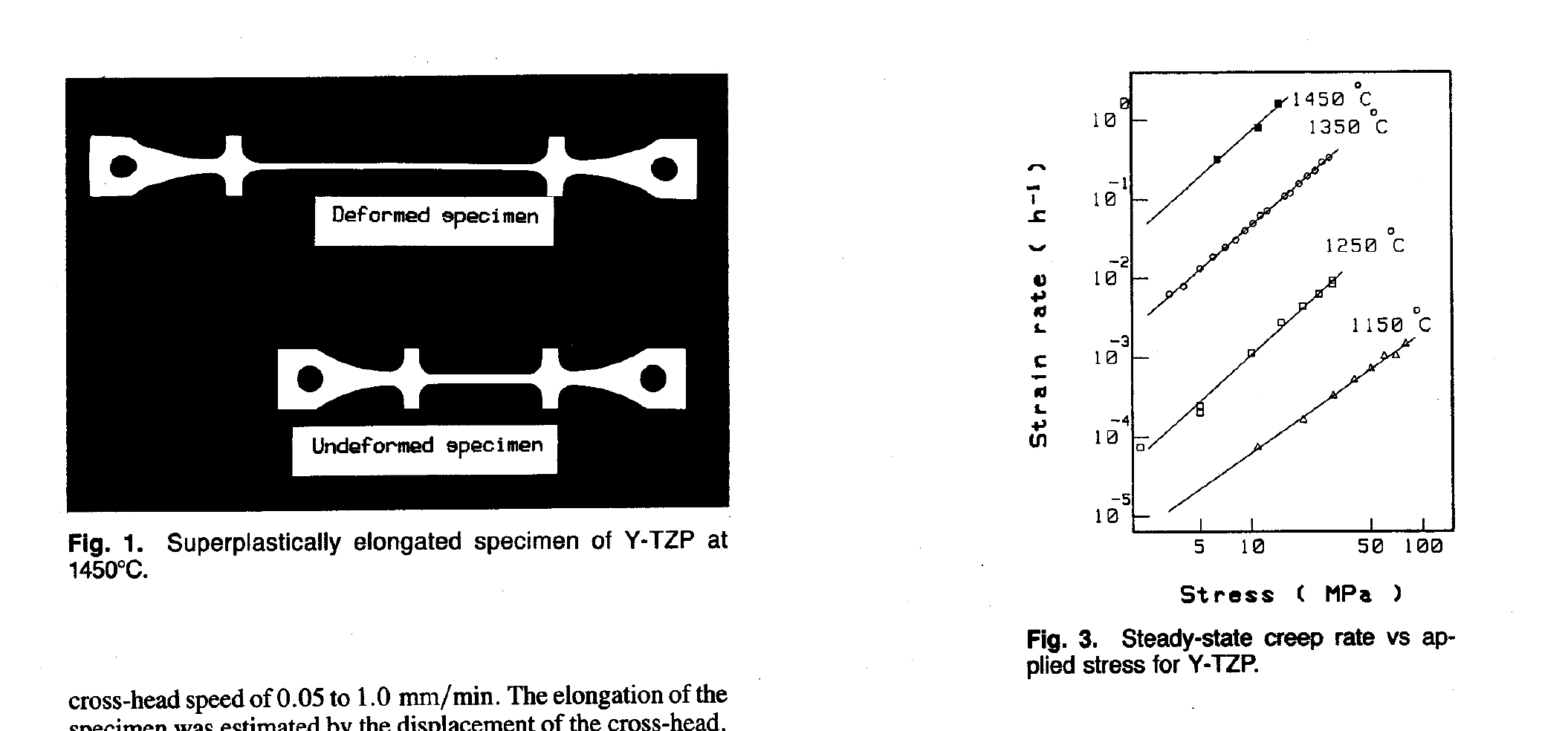
which is from this paper: Wakai in the late Eighties

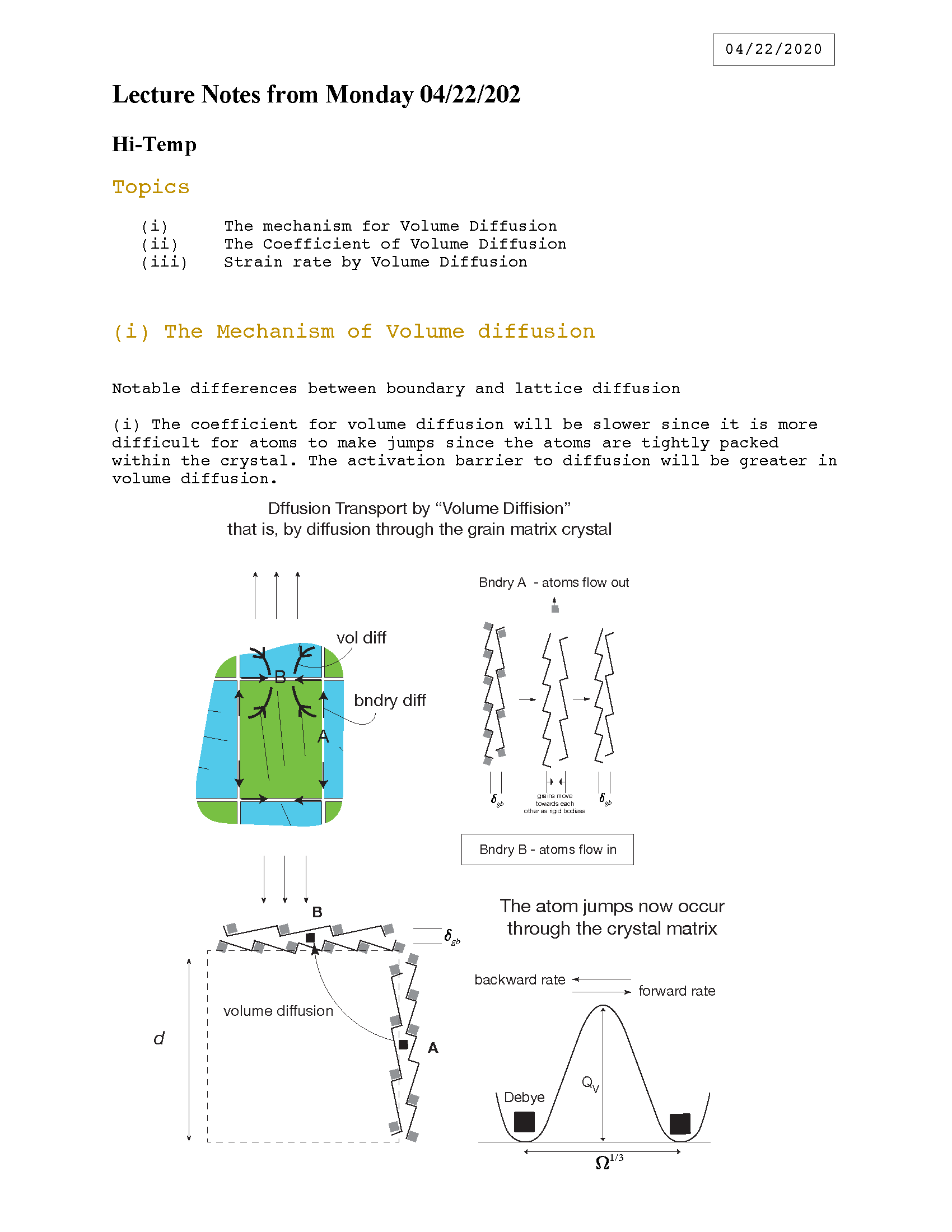
Strain Induced by Atom-by-Atom Transport of Mass Between Grain Boundaries

The notes from this class are bundled together in the following pdf file

Notes from April 15

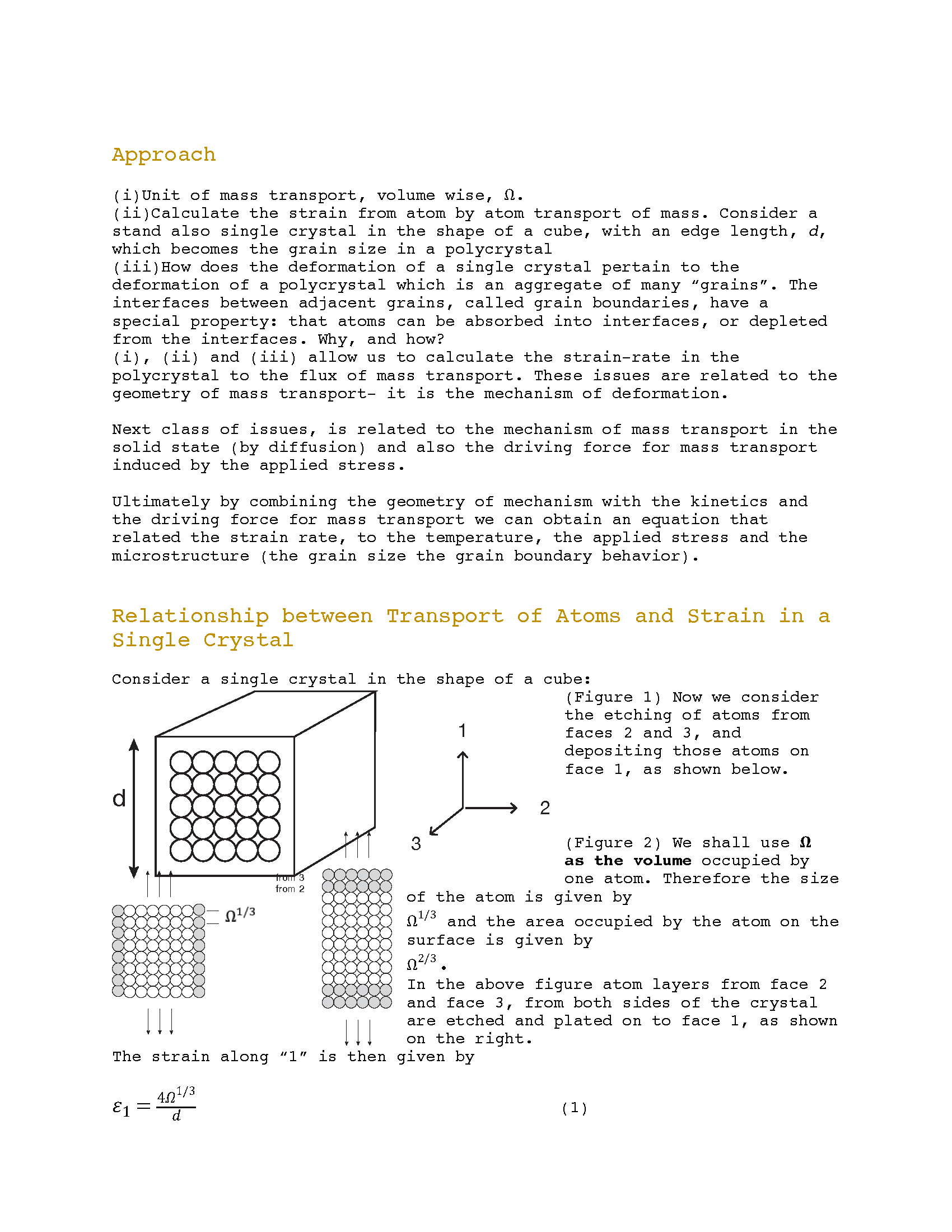
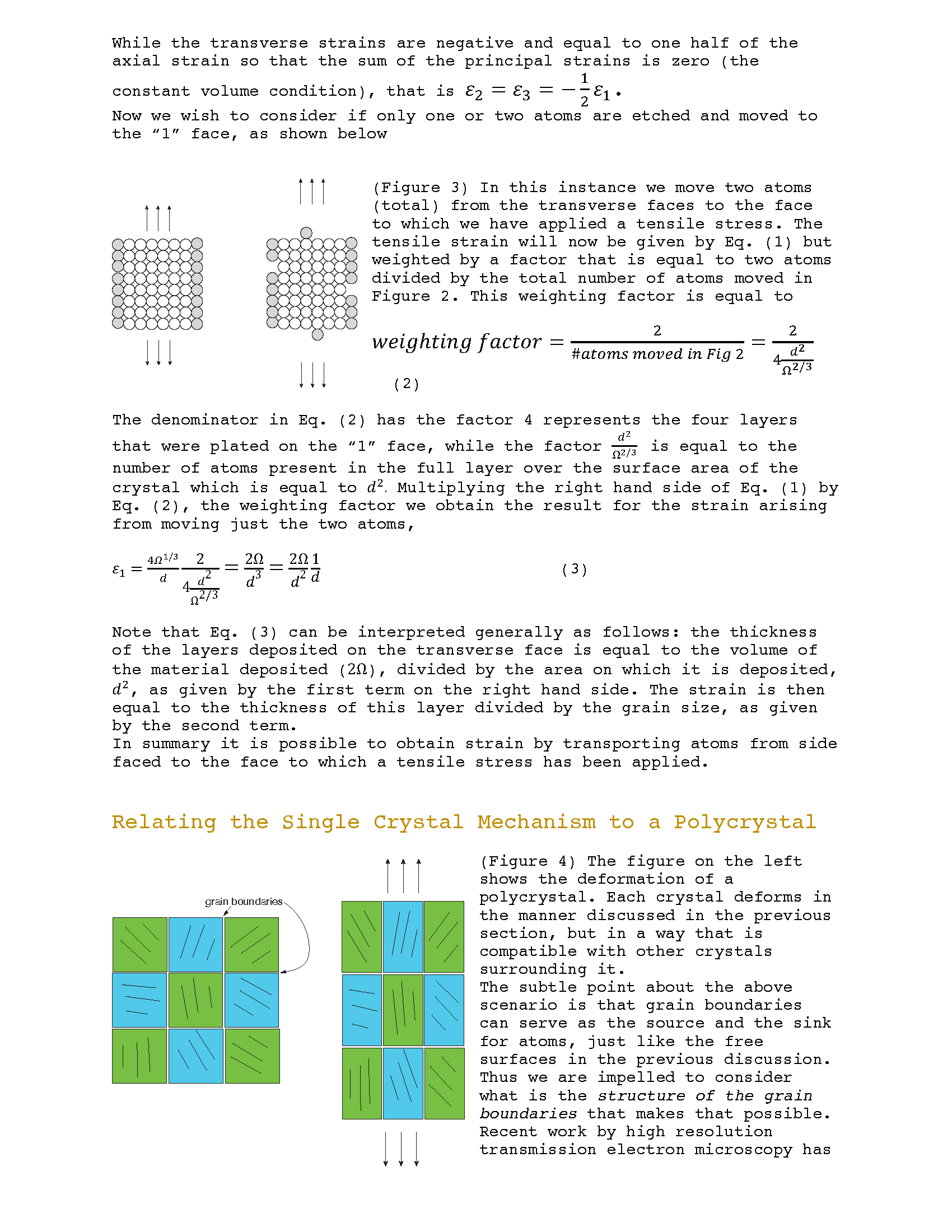
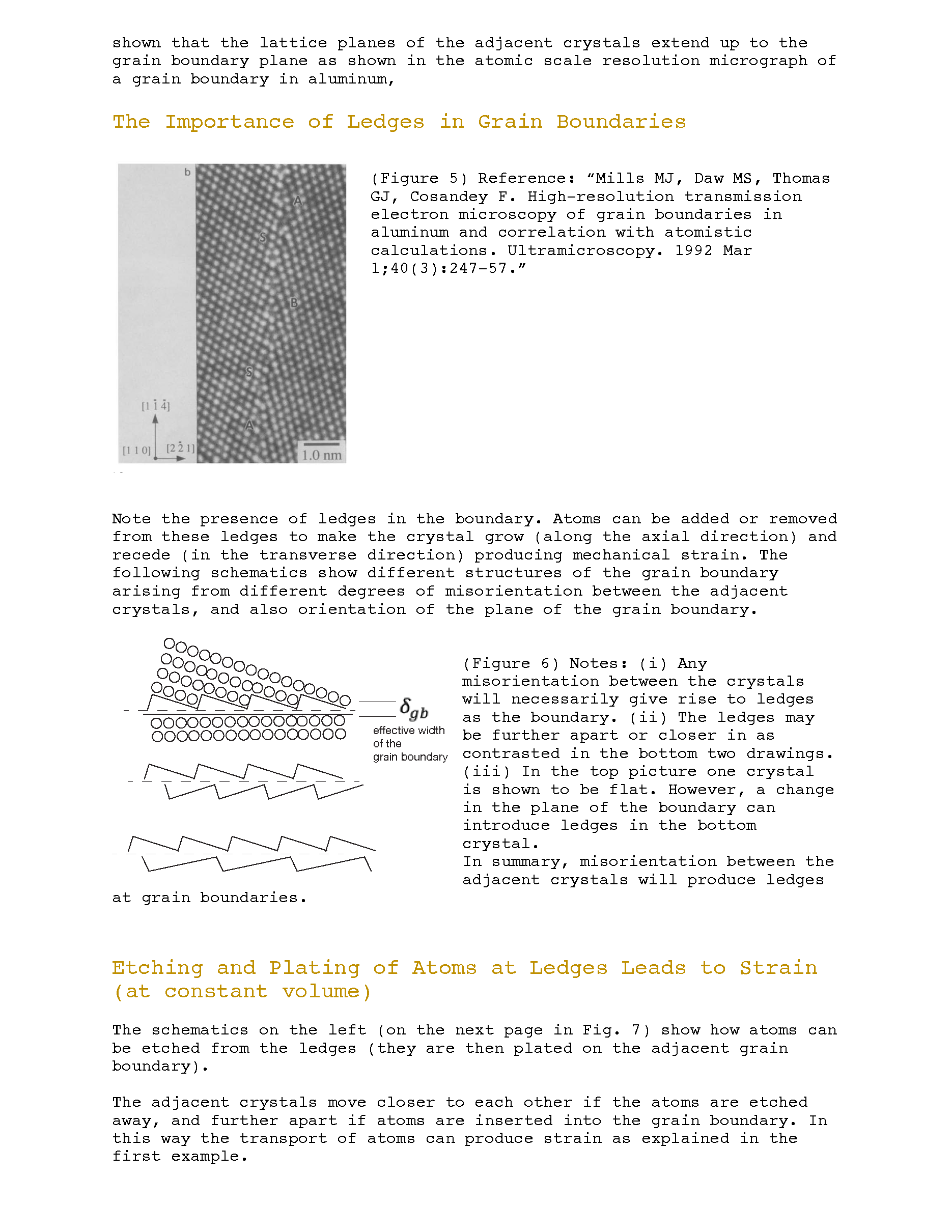
Strain Rate from Grain Boundary Diffusion

The notes from this class are bundled together in the following pdf file

Notes from April 20

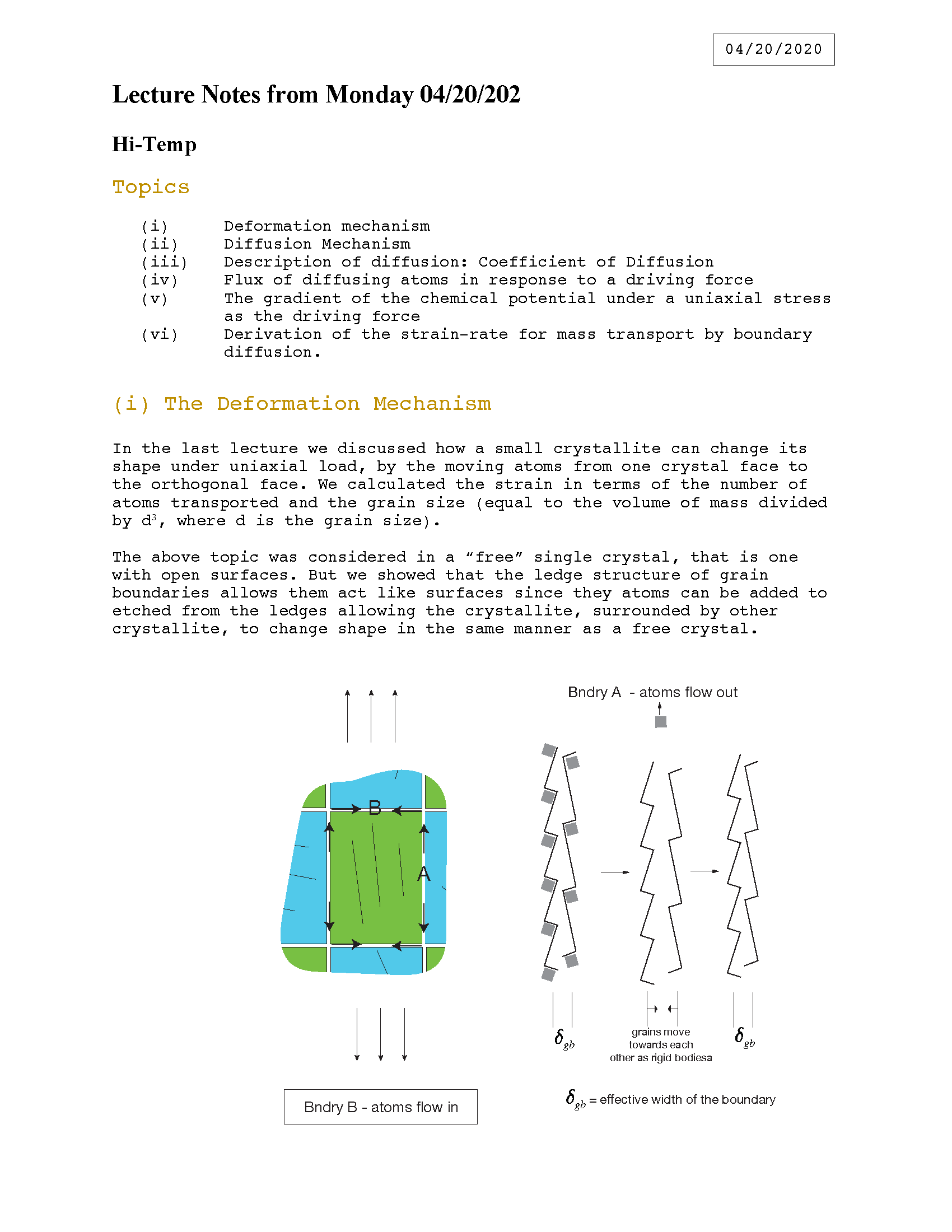
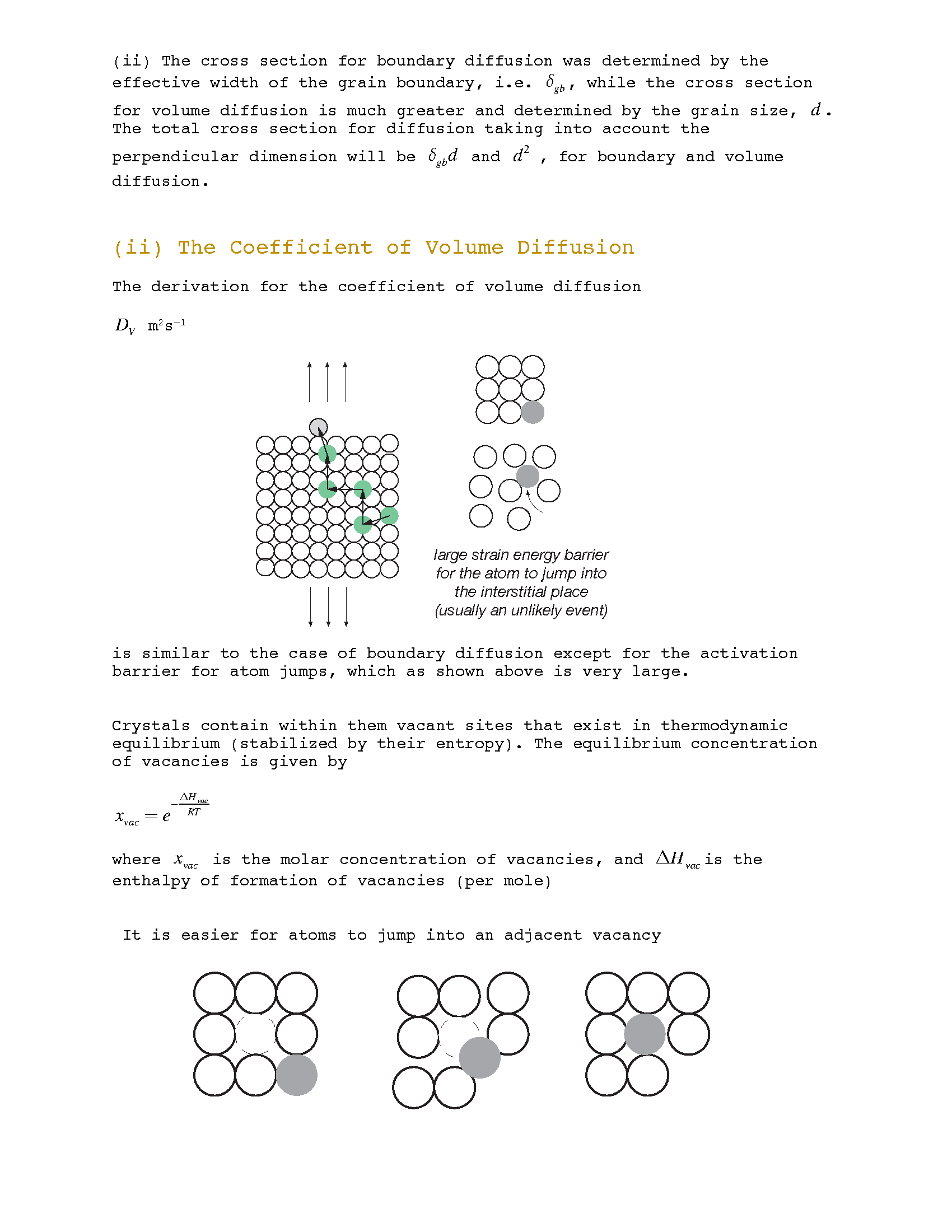
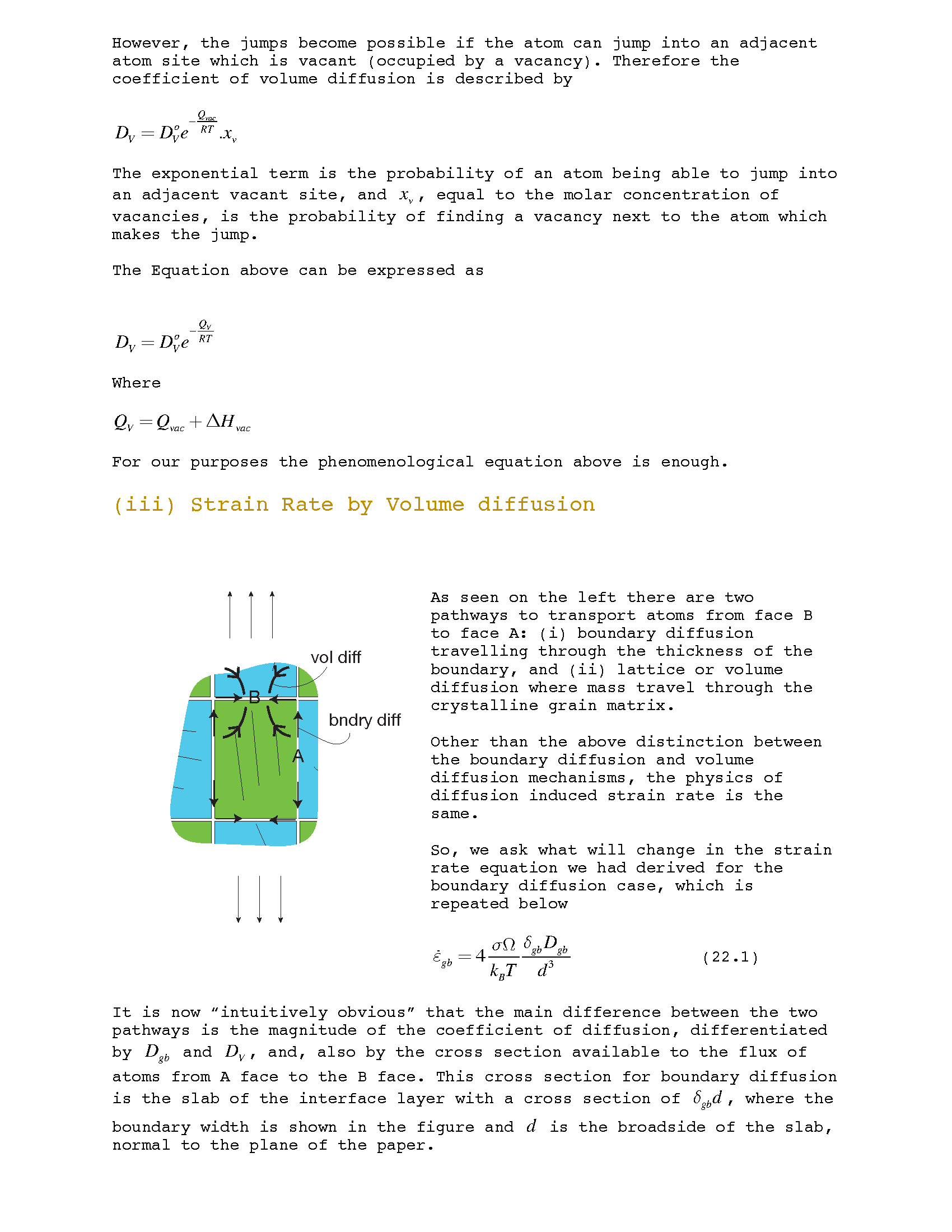
Strain Rate from "Volume" Diffusion

The notes from this class are bundled together in the following pdf file

Notes from April 22